NCERT Solutions for Class 8 Maths Chapter 3 Understanding Quadrilaterals Ex 3.2
Ex 3.2 Class 8 Maths Question 1.
Find x in the following figures.Solution:
(a) Sum of all the exterior angles of a polygon = 360°
125° + 125° + x = 360°
⇒ 250° + x = 360°
⇒ x = 360° – 250° = 110°
Hence, x = 110°
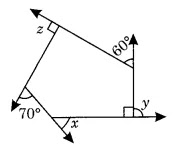
(b) Here, ∠y = 180° – 90° = 90°
and ∠z = 90° (given)
x + y +
60° + z + 70° = 360°
⇒ x + 90° + 60° + 90° + 70° = 360°
⇒ x + 310° =
360°
⇒ x = 360° –
310° = 50°
Hence, x = 50°
Ex 3.2 Class 8 Maths Question 2.
Find the measure of each exterior angle of a regular polygon of(i) 9 sides (ii) 15 sides
Solution:
(i) Sum
of all the exterior angles of a polygon = 360°
Measure of each angle of 9-sided regular polygon
= 360/9 = 40°
(ii) Sum of all the exterior angles of a polygon
= 360°
Measure of each angle of 15-sided regular
polygon = 360/15 = 24°
Ex 3.2 Class 8 Maths Question 3.
How many sides does a regular polygon have if the measure of an exterior angle is 24°?Solution:
Sum of
all the exterior angles of a regular polygon = 360°
Number of sides
Ex 3.2 Class 8 Maths Question 4.
How many sides does a regular polygon have if each of its interior angles is 165°?Solution:
Let n be the number of sides of a regular polygon.
Sum of all the interior angles of a polygon = (n – 2) × 180°
and, measure of its each angle
Ex 3.2 Class 8 Maths Question 5.
(a) Is it possible to have a regular polygon with measure of each exterior angle as 22°?(b) Can it be an interior angle of a regular polygon? Why?
Solution:
(a) Since the sum of all the exterior angles of a
regular polygon is 360°, which is not divisible by 22°.
So, it is not possible to have a regular polygon with measure of each exterior
angle as 22°.
(b) Sum of all interior angles of a regular polygon with
side n = (n – 2) × 180°
Since number of sides cannot be in fractions.
It is not possible for a regular polygon to have its interior angle as 22°.
Ex 3.2 Class 8 Maths Question 6.
(a) What is the minimum interior angle possible for a regular polygon? Why?(b) What is the maximum exterior angle possible for a regular polygon?
Solution:
(a) Sum
of all interior angles of a regular polygon with side n = (n – 2) × 180°
The measure of each interior angle
Therefore,
the minimum number of sides in a regular polygon is 3.
The minimum measure of the angle of an
equilateral triangle = 180°/3 = 60°.
(b) From part (a) we can conclude that the
maximum exterior angle of a regular polygon = 180° – 60° = 120°.
Related Links:
NCERT Solutions for Maths Class 9
NCERT Solutions for Maths Class 10
NCERT Solutions for Maths Class 11